I have briefly discussed mathematical Platonism in previous articles. This post is going to contain my current thoughts about it.
Mathematical Platonism is distinct from classic Platonism (so nothing about ideal forms, for instance) and holds that three propositions are true.
1. Mathematical objects exist.
2. Mathematical objects are independent of human beings.
3. Mathematical objects are abstract
Or, in condensed form, mathematical objects exist abstractly and independently of human beings. All possible mathematical objects exist, have always existed and will always exist, even if no mathematician ever ponders them.
In this post, I will argue that while there is no fact of the matter regarding whether mathematical objects exist, it is sensible and useful to treat them as if they do, and that this is enough to justify mathematical Platonism.
In this post, I will argue that while there is no fact of the matter regarding whether mathematical objects exist, it is sensible and useful to treat them as if they do, and that this is enough to justify mathematical Platonism.
Mathematical Platonism is not Mystical
To me, mathematical Platonism seems correct as long as we don't confuse the notion of abstract existence with physical existence. As discussed previously, there can be more than one kind of existence.
In particular, nobody is saying that mathematical objects are floating around in some ghostly mathematical realm of existence (even though I believe this is how Plato visualised it). Instead, my view is that it's a completely different category of existence, and if you disagree with me then you simply have a narrower concept of what the term "existence" means.
For example, it just seems to make sense to assume that when I say "There exists no rational square root of 3", the verb "exists" is meaningful and used appropriately. It is in this sense alone that mathematical objects exist. There really is nothing mystical about it, as much as some people perceive it this way.
It's not hard to see why this misconception about mathematical Platonism is so pervasive. The original motivation for positing the existence of mathematical objects was to explain by analogy to physical objects how mathematicians could perceive mathematical truths. It was supposed that mathematical objects were real, and could be perceived directly by the mind of mathematicians with some special kind of sense much as we see tables and chairs with our vision.
There were also ideas that we had originally come into the world from the realm of mathematics, and our perception of mathematical objects was some sort of memory of the things we had seen there before we had been born.
I don't think either of these ways of thinking about it make much sense, and this is not at all what I am proposing. In many ways it's unfortunate that the terminology of mathematical realism has become so inextricably bound to the philosophy of Plato, as much of his metaphysics don't really conform to the views of most modern mathematical Platonists.
Before I go any further, I would like to make clear that I don't actually think there is a single objectively correct answer to the question of whether mathematical objects exist. The idea of existence is dubious when applied to cases like this. It is at its most coherent when applied to physical objects in the universe such as the chair I am sitting on as I type. It seems without question that this chair exists. I can see it and touch it, and so could you if you were beside me. It is in contexts such as this that the concept of existence first arose.
There are many other kinds of entities which are not physical objects. Your mental experience, composed of thoughts, hopes and dreams is a good example. Though there may be physical analogues of these (e.g. the firing of neurons), it seems odd to suppose that the firing of the neurons is the same thing as the feelings they excite.
And yet few of us would deny that our minds or consciousness experiences are real. If this is so, it must be that these phenomena exist in some sense, although not in the same way that a chair does, as they are only accessible to ourselves.
Another kind of existence might be that of very high level emergent phenomena, such as nation states, languages, currencies, etc. Most of us feel that these things exist in a sense, even if they are only socially constructed. Multiple observers might agree that they exist, and yet nobody can perceive them directly. As such, it seems to me that whatever kind of existence they may have, it is not physical.
Examples abound in nature too. Photosynthesis is a process, not a physical thing. The idea of a species is a fuzzy high level descriptor which we apply to many different individuals. Genes are not single objects but patterns we find repeated in the cells of related organisms. All of these things have a basis in the physical, but they are not tangible objects, so they do not exist in the same way as a chair.
The idea of physical existence also breaks down when considering universes. Universes are not physical objects, they are the containers of physical objects. For a physical object to exist, it means that it must be present within the universe -- observers within that universe must be able perceive it or its effects to exist with their senses. The question of whether other universes exist is meaningless if we think of them as physical objects, because from our perspective they do not exist, as they are entirely causally disconnected from us. On the other hand, our universe doesn't physically exist from the point of view of an observer in another hypothetical universe.
And yet, most of us believe that the universe exists!
So we ought to be open to the idea that there are things other than the physical, including mathematical objects. Whether the concept of existence is applicable in all cases is a matter for only for linguistic definition. Clearly, each of these objects, processes and properties has an existence in some sense, even if not in the same way that a chair does. There is no fact of the matter - whether this pseudo-existence is real or not depends only on how one defines existence. Definitions ought to be judged on how well they capture intuitive concepts, on how consistent they are and on how useful they are for communication, and it is on these grounds that we should decide whether mathematical objects exist or not.
For me, there are two particularly striking arguments that convince me that mathematical Platonism is a useful and consistent way to think about mathematical objects.
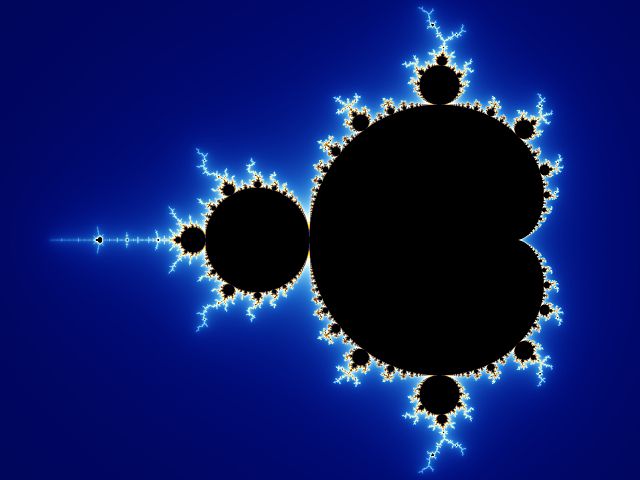
Entities such as the Mandelbrot set and other such visually striking mathematical objects are particularly good demonstrations of the intuition that mathematical concepts exist. The Mandelbrot set is defined by deceptively simple mathematical rules. I imagine that Benoit Mandelbrot, the mathematician who first stumbled upon these rules, found himself surprised by the complexity and beauty he saw unfolding before him as he explored the consequences of his simple premises (and even if he were more sanguine, I can certainly imagine being pretty excited if I were in his place).
We see the same kind of thing in Conway's Game of Life, a simple mathematical system which turned out to have exciting and profound consequences and phenomena to be explored. Many hobbyists and mathematicians are still exploring this and other systems like it. Mathematician Stephen Wolfram (creator of the well-known Mathematica mathematics package) published a book in 2002, A New Kind of Science, all about exploring the kind of complex and surprising phenomena we can find emerging from even simpler such systems.
If the "inventor" can be genuinely surprised by his own "invention", then it seems to me that he is discovering truths about it that he did not put there himself. In my view, this can only be consistent with the view that this really is exploration of something that exists independently.
We see the same kind of thing in Conway's Game of Life, a simple mathematical system which turned out to have exciting and profound consequences and phenomena to be explored. Many hobbyists and mathematicians are still exploring this and other systems like it. Mathematician Stephen Wolfram (creator of the well-known Mathematica mathematics package) published a book in 2002, A New Kind of Science, all about exploring the kind of complex and surprising phenomena we can find emerging from even simpler such systems.
If the "inventor" can be genuinely surprised by his own "invention", then it seems to me that he is discovering truths about it that he did not put there himself. In my view, this can only be consistent with the view that this really is exploration of something that exists independently.
I also like to bring up the idea of independent discovery by separate people of the same mathematical object, such as the discovery by Newton and Leibniz of the calculus. The controversy which arose over which of them had first discovered it is only sensible if we view it as discovery. If a mathematician is like a cabinet maker, creating something new whenever he invents a new mathematical tool, there would be no issue. Newton would have his cabinet and Leibniz would have his. The fact is that they both discovered the same thing, independently, like two explorers happening upon the same continent. This to me demonstrates that mathematical objects are independent of mathematicians. It also suggests that they exist, as otherwise there would be nothing for the two to discover independently, and so nothing to argue about.
Now, admittedly, these two arguments I have sketched out are essentially arguments from intuition, and intuition can be very fallible indeed. I think this is not a problem in this case precisely because I maintain that there is no fact of the matter. "Existence" in its general sense is simply not a robust, objective concept we can all agree on, like mass or velocity. Instead, it's just a label for a human intuition. As such, we ought to apply it in cases which make the most intuitive sense, as long as we're not contradicting ourselves or the evidence before us.
The arguments from intuition I have presented I justify the utility and coherence of thinking of mathematical objects as existing.
Since I think ultimately any disagreement must boil down to differences of interpretation of the word "existence", this is arguably a question only of semantics. As such, it may appear to be completely inconsequential and so irrelevant to any serious issues, but I feel it actually has profound implications for how we explain certain other phenomena we perceive as real.
The human mind, for instance, does not appear to be a physical object in itself, but is instead some sort of process which takes place in a human brain. If the computational theory of mind is true, then the human mind is a computational process, which is a kind of mathematical object. Whether the computational theory of mind is right or wrong, it is at least plausible enough to convince a lot of people, including me. If you accept that it is at least plausible, then what would it mean for the existence of the mind if it were true?
Rejecting mathematical Platonism would mean that it is plausible that the human mind does not exist, or that it is an illusion or fiction. If we instead allow ourselves the mental tool of mathematical Platonism, then we get to accept both the computational theory of mind and that the mind exists. Whichever approach you choose should depend on which definition of existence leads to the most intuitive conclusions, and I think most of us prefer to think that our minds are real (even if not in the same sense as tables or chairs).
I think the same is true of the universe. As I explained before, the concept of physical existence doesn't really make sense when applied to universes. We can't really say that our universe objectively exists because we all have the special, subjective viewpoints of observers within it. What about other hypothetical universes? What about the fictional universe of Star Trek, for instance? It does indeed exist from the point of view of Captain Kirk, and there is no way he could tell that he or his universe do not really exist. It seems to me that we have no robust argument to show that our own universe is any more real than that of Star Trek's.
One relatively popular instance of this kind of view is Nick Bostrom's simulation argument, where he proposes that we could all be living in a computer simulation. Computer simulations are of course computational processes, which are a special kind of mathematical object.
If we imagine for a moment that this is in fact the case, then a denial of mathematical Platonism would amount to a denial that the universe exists, while allowing ourselves the mental tool of mathematical Platonism allows us to consider this possibility while maintaining that the universe would actually exist even if it were in fact a computer simulation. Again, whether we accept or reject mathematical Platonism should therefore be predicated on which definition of existence allows for the conclusion that makes the most intuitive sense.
So if you reject mathematical Platonism, that's fine. But if you do, and in particular if you are willing to assign a non-zero probability that a computer could have a mind or that the universe could be a simulation, then you ought to be ready to entertain the idea that you, your mind and the universe around you may not actually exist in any sense.
I prefer mathematical Platonism.
The human mind, for instance, does not appear to be a physical object in itself, but is instead some sort of process which takes place in a human brain. If the computational theory of mind is true, then the human mind is a computational process, which is a kind of mathematical object. Whether the computational theory of mind is right or wrong, it is at least plausible enough to convince a lot of people, including me. If you accept that it is at least plausible, then what would it mean for the existence of the mind if it were true?
Rejecting mathematical Platonism would mean that it is plausible that the human mind does not exist, or that it is an illusion or fiction. If we instead allow ourselves the mental tool of mathematical Platonism, then we get to accept both the computational theory of mind and that the mind exists. Whichever approach you choose should depend on which definition of existence leads to the most intuitive conclusions, and I think most of us prefer to think that our minds are real (even if not in the same sense as tables or chairs).
I think the same is true of the universe. As I explained before, the concept of physical existence doesn't really make sense when applied to universes. We can't really say that our universe objectively exists because we all have the special, subjective viewpoints of observers within it. What about other hypothetical universes? What about the fictional universe of Star Trek, for instance? It does indeed exist from the point of view of Captain Kirk, and there is no way he could tell that he or his universe do not really exist. It seems to me that we have no robust argument to show that our own universe is any more real than that of Star Trek's.
One relatively popular instance of this kind of view is Nick Bostrom's simulation argument, where he proposes that we could all be living in a computer simulation. Computer simulations are of course computational processes, which are a special kind of mathematical object.
If we imagine for a moment that this is in fact the case, then a denial of mathematical Platonism would amount to a denial that the universe exists, while allowing ourselves the mental tool of mathematical Platonism allows us to consider this possibility while maintaining that the universe would actually exist even if it were in fact a computer simulation. Again, whether we accept or reject mathematical Platonism should therefore be predicated on which definition of existence allows for the conclusion that makes the most intuitive sense.
So if you reject mathematical Platonism, that's fine. But if you do, and in particular if you are willing to assign a non-zero probability that a computer could have a mind or that the universe could be a simulation, then you ought to be ready to entertain the idea that you, your mind and the universe around you may not actually exist in any sense.
I prefer mathematical Platonism.
Hi Disagreeable,
ReplyDeleteThanks for another interesting post. I guess you won't be surprised to hear that I disagree with some of it.
"Mathematical Platonism Is True Because it is Useful"
As far as I'm concerned it's not mathematical platonism that's useful. It's mathematics that's useful.
My objection to mathematical platonism is that I think its talk about the existence of mathematical objects is confused and confusing. It's much better to talk about the truth of mathematical statements. I'm on board with the fact that mathematical statements are (or can be) pure abstractions and that the truth of mathematical statements is observer-independent.
I have no problem with the mathematician's talk of existence, e.g. "there exists an integer between 2 and 4". That makes sense as a true statement of an axiomatic system. But when you step outside of any axiomatic system and talk about the existence of mathematical objects in a more general sense, such talk ceases to have any meaning. The mathematician's talk of existence is useful. The mathematical platonist's is not.
"The human mind, for instance, does not appear to be a physical object in itself, but is instead some sort of process which takes place in a human brain. If the computational theory of mind is true, then the human mind is a computational process, which is a kind of mathematical object."
This sounds to me like rather loose hand-waving: a mind is kind of a computational process, and a computational process is kind of related to mathematics, so a mind is kind of a mathematical object. I suggest you need to think more carefully about what you mean by "mathematical object". As far as I'm concerned, if "mathematical object" is to mean anything useful it must refer to something that can appear in a pure mathematical statement, like a number, set, etc. I don't see how a mind can be taken in that way.
Human minds are instantiated in real physical systems (brains). I would say that they supervene on those systems. They are not pure abstractions. Even if we talk about minds in a very general way (without thinking about any particular instantiation) our statements about minds are very different from the statements of axiomatic systems.
I would suggest that we have to be careful in talking about abstractions. As far as I'm concerned all our statememts are abstractions to some degree. We model reality at various levels of abstraction. Our models of the mind are particularly abstract, but I wouldn't draw a fundamental divide between our models of physical objects and our models of mental objects (like beliefs and desires). (I'm not addressing consciousness here.) Our use of the word "physical" to describe the former but not the latter should not be attributed too much significance. Pure mathematical statements are, however, different from both, in that they are purely abstract. So mind-talk is more like physical-world-talk than like pure mathematics. It's a mistake to jump from "minds are abstract" to "minds are the same sort of thing as mathematical objects".
Hi Richard,
DeleteOnce again, thank you for your detailed and thoughtful response.
I agree that mathematical Platonism is not quite as useful as mathematics. I do think it's useful in allowing us to build a coherent, consistent picture of reality, but it's not going to be helping us to build bridges any time soon. As such, it's only useful to philosophical enterprises such as understanding consciousness or why the universe exists.
I can understand why you think that talk of mathematical objects as existing or not is in some way incoherent. It isn't though if you just interpret "exist" in the same sense as the hypothetical mathematician you quoted. My point is that if the mind is a mathematical object, and if the universe is a mathematical object (both statements I believe but you do not), then this is a useful way to think of how it is that these exist.
I wouldn't say a computational process is kind of related to mathematics, I would say a computational process really is a mathematical object.
I would say a mathematical object is more than something that can appear in a mathematical statement. A statement is itself a mathematical object, and so is any abstract object or system that can be analysed mathematically. Some examples:
The Cartesian plane
Peano arithmetic
An equation
A parabola
The natural numbers
The set of all mathematical objects
A sequence of characters (and so any block of text)
A function
An algorithm
A computational process is an instantiation of an algorithm. Now, I recognise that there appears to be a distinction between an algorithm and its physical instantiation, and indeed I have done some hand-waving here. However I hope to explain in future why it is that the process itself can also be regarded as an abstract mathematical object. For now, you can take as a simple argument the idea that the character of the computation is substrate independent, that the mind would not be meaningfully altered if these computations were carried out by electronic rather than organic matter.
The mind can also be thought of as the function that maps input nerve signals to output nerve signals.
In any case, my purpose in this post is not to convince you that the mind is a mathematical object. Instead, I'm arguing that if I can convince you that the mind is a mathematical object, then mathematical Platonism is useful in reconciling this with our belief that our minds exist. It also helps us to understand the virtual minds response to the Chinese Room, and how it can be that matter can have phenomenal experience.
I'm with Richard, I'm afraid. I have never encountered a mathematical object that wasn't instantiated in a real physical system. I have an intuition that what seems abstract is simply the human mind doing what it's so good at - recognising patterns. In other words, being intuitive. Now, there's a paradox for you :)
Delete(Steve Morris)
Hi Steve,
DeleteThanks for your comment.
Of course you have never encountered a mathematical object that wasn't physically instantiated - the act of perceiving such an object would mean it is physically instantiated in your brain in some form.
But that doesn't explain how we ought to view independent discovery or how it is that the very same mathematical concept can have very different physical instantiations and still be held by you to be identical to those disparate physical instantiations.
Just my point of view: It appears to me that a constructive, programmatic version of MUH (CUH or PUH, which Tegmark seems to really advocate from what I've read) can be embraced by a self-defined anti-Platonist (like Solomon Feferman, who just turned 85!). Is that enough for science? Who knows.
ReplyDeleteHi Philip,
DeleteI don't know anything of Solomon Feferman.
I think any variant of the MUH/CUH which does not embrace Platonism faces a couple of problems
How is it that the universe exists? Why is it fine-tuned?
Platonism answers both questions because in Platonism all mathematical objects exist.
If the set of programs can include hyperprograms or transfinite programs (http://www.hypercomputation.net), then that set might express all possible mathematics.
DeleteFair enough, Philip, but now you sound like a programmatic Platonist though you deny mathematical Platonism.
DeleteI do feel that all possible programs exist. Do you also? If so, then why do you feel mathematical Platonism is wrong?
Had a few thoughts on this, though I appreciate it's from a while ago. (Already wrote one reply but it got wiped when I logged in to post, bah!)
ReplyDeleteYou seem to be arguing for mathematical platonism on the basis that propositions like "there exists a prime > 8" uses the term ‘exists’ in an appropriate way (presumably because it's true, and it's hard to see how a term used in a true statement could be being used inappropriately).
Now, I think the word 'exists' is being used perfectly appropriately there, but I am not a mathematical Platonist. I understand Platonism as the view that there exist real entities to which the terms in mathematical propositions refer (for example the numeral ‘8’ in the above example refers to the entity: 8), and it is the real relations between these real entities which account for mathematical propositions being true or false - i.e. we can take them literally. (These real entities need be analogous with, say, physical entities, only insofar as we can apply the word ‘real’ to both— I would say that’s the minimal condition on being a Platonist.)
A constrasting picture would be offered by something like nominalism, which holds, roughly, that a the number 8 is just the set of real entities which instantiate certain properties — the additive and multiplicative properties we associate with ‘8’. This differs from Platonism in several respects:
1. There are no 1-to-1 mappings between mathematical terms (like the numeral ‘8’) and real entities, though there may be 1-to-many mappings.
2. Such relationships, if they exist, need not exist: the set of real entities instantiating 8 may not have existed, in which case 8 would not have existed. 8 does exist, but an incomprehensibly large number, like, say, Graham’s number to the power of itself, does not exist.
3. Mathematical propositions are true not in virtue of real relations of real entities, but of possible relations between sets of possible entities. So the proposition "there exists a prime > Graham’s number to the power of itself" is true, not because there is a set of real entities instantiating that property (there isn’t, it’s too big), but because there might have been.
OK, so none of that is likely to sell nominalism, but I do think it illustrates the contrast between the sort of thing that Platonism wants to say and the sort of thing that anti-realist theories of mathematics which take mathematics seriously want to say.
Perhaps you’re using Platonism in a broader sense than I am, in which case that will all ring hollow for you, but then the question can just be rephrased in terms of what sort of Platonists we are. Make any sense?
Sam
Hi Sam,
DeleteThanks for commenting.
If you believe that it is appropriate to say that a mathematical object exists, but that there is not necessarily a real entity which matches that object, is this not equivalent to saying that it is possible for something to exist but without it being a real entity? To me, this is a contradiction, because an entity is just something that exists.
Or perhaps you would say there is such an entity, but it is potential rather than real? OK, but to me it's just a rephrasing of the question -- now instead of asking what exists we are asking what is real. To me, those are essentially the same question. I view mathematical objects as real because it is useful to do so. There is no fact of the matter on whether they are real or not because it depends only on how you define reality.
As with free will anti-realism and compatibilism, I view the question of mathematical realism as semantic only. I don't think there is a profound distinction between the metaphysics of nominalism and Platonism, in the sense that both approaches can be valid at the same time. However it does seem that the two attitudes entail profoundly different ways of thinking about mathematics.
And there are other reasons to prefer Platonism. For various reasons explained elsewhere on this blog I think the mind is a mathematical object and also that the universe is a mathematical object. Ultimately I am a mathematical monist. If my metaphysics are right (which most people will surely deny), then it turns out that nominalism is semi-incoherent and Platonism is required if we want to say anything exists at all.
So the point of this post is to dispel the idea that there is anything mystical about Platonism. Mathematical objects are not ghostly forms floating around in a void on some other plane of existence. It's just an attitude about what we consider real. With this clarified, I hope to build arguments for the broader metaphysical view.
Hi, thanks for your reply.
Delete“So the point of this post is to dispel the idea that there is anything mystical about Platonism. Mathematical objects are not ghostly forms floating around in a void on some other plane of existence. It's just an attitude about what we consider real. With this clarified, I hope to build arguments for the broader metaphysical view.”
I agree that there’s nothing mystical about Platonism - I think there some good prima facie arguments for Platonism, though I think ultimately they fail in the face of its problems. However, Platonism does entail that there are such things as abstract entities which are not physical (nor, on some accounts, causally active). These don’t need to couched in material terms like ‘ghostly’ and ‘floating’, but they need to exist for Platonism to be true.
Jumping to the top:
“If you believe that it is appropriate to say that a mathematical object exists, but that there is not necessarily a real entity which matches that object, is this not equivalent to saying that it is possible for something to exist but without it being a real entity?”
There's nothing contradictory here - it’s to say that ‘exists’ as used in the mathematical context doesn’t depend on general philosophical theories of existence. (Hence why mathematicians don’t need to care about the mathematical realism debate to keep doing what they do.)
This is already illustrated when you say “I view mathematical objects as real because it is useful to do so.” When a mathematician says “there is a prime number > 8”, they are not saying “it is useful for me to believe that there is a prime number > 8”, they are just saying that there is one. So here what you’re offering is an instrumental criteria for mathematical truth, and by proxy the reality of mathematical entities. This is fine, but it most certainly isn’t Platonism.
Or perhaps the point is more general: perhaps all it means for anything to exist is that it is useful to view it as if it does. This ‘global instrumentalism’ is something I have deep sympathies with, but it doesn’t really help us with the debate over mathematical realism. All it does is change the question from ‘do mathematical entities exist?’ to ‘in what sense are existential statements in mathematics useful?’
Things can be useful because they represent the world (e.g. maps), or for other reasons (e.g. hammers). From the point of view of global instrumentalism, to ask whether mathematical Platonism is true is to ask whether mathematical truths (including existential truths like “there exists a prime > 8”) are useful in virtue of representing the world, or in virtue of something else. So the question being debated by philosophers of mathematics is left largely untouched by this line of thought.
The other thing about instrumentalism is that even if true, it does not mean that it is "just an attitude about what we consider real." In particular, if to be real is to be useful, then what is real is non-arbitrary, because it is constrained by what is useful.
Sam
Hi Sam,
DeleteI'm not trying to say that mathematical objects are real because they are useful -- I don't think utility has much to do with reality. Plenty of mathematical objects are not at all useful and that makes them no less real.
What I am saying is subtly different. I am saying Platonism is correct because it is useful to use the language of existence when discussing mathematical objects. A nominalist mathematician says "there exists a prime > 8" but when pressed might admit that she is using the word "exists" in a metaphorical or fictional sense. A Platonist says there is no need for such caveats. It is perfectly reasonable to say mathematical objects actually exist and are actually real as long as we do not narrowly equate reality or existence with physicality.
The question of whether they are real or not is simply unanswerable, because it is meaningless unless you specify what kind of reality you're talking about. If you prefer a narrow definition, that's fine, but if I'm right about the philosophy of mind and the nature of the universe that means that neither of us are real and neither of us exist. If you think that's OK, then that's fine by me.
Right, that is a different argument (actually it's what I was talking about when I mentioned there being prima facie arguments for Platonism). Indispensability arguments (noting that you need not just that existence is useful in maths, but also that maths is useful in general) of that type are valid, but ultimately not that strong, in my view. They offer a prima facie case, so in the absence of objections they would be enough. But there are loads of objections to mathematical Platonism (the argument from epistemological access is particularly damning). I wrote something about this recently, so won't go into it at length (in case you're interested, it's quite short: http://theplatopus.com/2014/07/12/on-the-indispensability-of-mathematical-objects/)
DeleteDon't really have any comments on your final paragraph, as it looks like there's a whole network of other stuff going on there which I don't want to guess at.
Sam
I read your article earlier. I didn't comment because I don't have any strong views on it. I don't regard my position as being based on indispensability arguments. In fact I find indispensability arguments quite weak for the same reasons you do.
DeleteRather my point is that it is a mistake to think that reality is a well-defined concept. It simply isn't the case that there is a correct answer to the question of whether mathematical objects are real, because "real" means different things to different people. The whole debate is the result of an evolved intuition that turns out to be too vague and/or ambiguous to be useful until clarified.
Since even nominalists find themselves using the language of existence when discussing mathematical objects, it seems to me there is no reason not to define "reality" so as to include them.
I know you have are not familiar with my thoughts on the philosophy of mind or the universe, so I'll put it this way. It is sometimes suggested that the universe might be a simulation. If this were true, would it follow that it does not exist or it is not real? We might say so, but we might also say that it is real and what it is is a simulation, that I exist and what I am is a virtual person. Since the meaning of "real" is up for grabs, I think it is more intuitive to adopt a definition where I can say with confidence that I am real and I exist, whether or not I am in a simulation. Otherwise, "cogito ergo sum" would not follow.
"Rather my point is that it is a mistake to think that reality is a well-defined concept. It simply isn't the case that there is a correct answer to the question of whether mathematical objects are real, because "real" means different things to different people."
DeleteI agree that reality is not well-defined. The point of ontology is, as I see it, is to map out the relationships between all the areas in which we tend to take things to be real: mathematical, physical, moral, whatever. As Wilfrid Sellars put it, "the task of philosophy is to articulate how things in the broadest sense of the term hang together in the broadest sense of the term." A successful ontology will unify the concept of the real, or argue for its intrinsic fragmentation. You can grant reality to anything which people call 'real' if you like, but this doesn't give you a unified concept of the real. It gives you lots of different concepts of the real with none of the links between them articulated, which is what we had in the first place anyway.
So when we discuss whether mathematical entities exist, what we are doing is asking how our usage of 'exists' in those cases relates to our usage of 'exists' in all the other cases in which it's used. Broadly, to be an anti-realist is to say that it doesn't relate in any substantial way, and that the other, non-abstract cases are more paradigmatic examples of the real. So the debate over mathematical Platonism doesn't rely on any pre-fixed notion of the real, and neither is it an arbitrary ascription (we can ascribe it arbitrarily, but this is to miss the issue). The real is rather a deeply primordial concept whose content is articulated dialectically.
Sam
Right, so the real is a deeply primordial concept, rooted I would say in evolved intuitions. This means that it is not necessarily a terribly coherent or well-thought out concept. If it is a concept we wish to make robust, we must define it so as to include objects it is useful to regard as existing and exclude others.
DeleteThere are plenty of ways in which abstract objects are similar to physical objects. They can be thought about. They can be explored. We can discover things about them by studying them. We can build a body of knowledge about them. We can use them as tools for practical purposes. Like places or substances, different people can discover them independently.
The number of similarities is such that I think it sensible to class as real all objects with these properties, both abstract and physical. This includes even fictional characters and contradictions.
For instance, there really does exist a character called Sherlock Holmes, but that is not to say that there is a physical person called Sherlock Holmes. There exist contradictory descriptions of objects such as "square circles" or "greatest prime", but there are no such actual objects. I don't think you can ever really think about the greatest prime, I think you are thinking about the concept of a greatest prime, which is a role that is not filled by any object. If you could really think about the greatest prime itself, that would mean you could explore it, for example discovering what its final digit is. But you cannot, you can only imagine what its final digit is, in which case you are simply amending the contradictory description that is the actual object of your ruminations.
"Right, so the real is a deeply primordial concept, rooted I would say in evolved intuitions. This means that it is not necessarily a terribly coherent or well-thought out concept. If it is a concept we wish to make robust, we must define it so as to include objects it is useful to regard as existing and exclude others."
DeleteI agree with this. However, I do not think the rest of your reply pays enough heed to the constraining role which 'useful' plays in the final sentence.
You give 3 criteria under which we could call mathematical entities real:
1. they can be thought about
2. we can discover things about them
3. we can use them as tools
(I've taken explorability to be the same as discoverability --- I hope that's fair, correct me if not.)
Working backwards, I don't think it's right to say that we use mathematical entities as tools. We use mathematical truths --- theorems and formulae -- as tools, and the question is whether we need to posit mathematical entities to account for mathematical truth (whether this truth is cashed out in terms of utility or not).
To say that we discover things about mathematical entities seems to me to just beg the question against mathematical anti-realists, who would say that mathematics is not discovered at all, but created. (With the nominalists adding that this creativity is constrained by features of the world, and fictionalists adding that this creativity is constrained by features of us as creators).
That leaves us with ‘can be thought about’, which seems to me to be way too broad to be useful. (On a side-note, if this is all it takes for something to be real, then on what grounds can we say that libertarian free will does not exist?)
The example of fictions like Sherlock Holmes illustrates this. Say we take all fictions to be real, then we ask the question: was Lao-Tzu a real person? Some think that he was, others think he was invented to impose some narrative unity on a heap of disparate ancient Chinese sayings.
This is clearly a substantial dispute (i.e. there is a fact of the matter), but if we take all fictions to be real, we can’t make good on it by asking whether Lao-Tzu was real or not. Instead we have to ask something like “was Lao-Tzu real in the sense of being a fictional person, or was he real in the sense of being a historical, physical person?” In other words, treating fictions as real does nothing to alleviate any dialectical tension — it just forces the same old problems to be translated into new (cumbersome) language. And this is why it fails on utility.
You said earlier in the thread that you thought utility was irrelevant (I’m not quite sure at this point where you stand on that point), so maybe all of the above will seem off-point. But then the question remains of how we can make the concept of the real robust?
Sam
Hi Sam,
DeleteFirstly I must apologise if I appear to be contradicting myself and jumping between all kinds of contrasting positions. Let me try to clarify a few things.
Utility is important because I regard Platonism as useful: it gives us the mental framework to discuss mathematical objects intuitively and is potentially important in resolving other questions, e.g. in philosophy of mind. The utility of mathematical objects themselves is not important. I hope you see the distinction. Since what exists is ambiguous, we should choose a useful definition, which is not necessarily to say that only useful things exist.
Perhaps we don't need mathematical entities as a way to explain the usefulness of mathematical tools. I didn't say we did. I'm just noting some similarities between some things which exist (including usefulness as tools) since you want the concept of existence to be unified. But we can drop this point because you're right that we don't directly use mathematical objects to do anything.
I think I would stand firm on the view that we discover things about mathematical objects, because we are constrained in what we can learn (and in ways which I think cannot be explained by features of the world or limitations of imagination). We can't, for example, find any arbitrary shape we want in unexplored regions of the Mandelbrot set. There are little corners of it that have never been seen by human eyes, but if I am the first to inspect one I am not free to create whatever I like there. We start out with a simple definition of a function (which you may view as an act of creation), but where we take it from there is very much an act of exploration -- we are exploring what that function entails and it may well surprise us. So exploration and creation do not have to be mutually exclusive.
Although I think interpreting mathematical objects as created is problematic. I think any two mathematical objects which are isomorphic are the same object -- my number two is the same as your number two. If two mathematicians discover the same thing independently (as Newton and Leibniz did with calculus, for example), then it's hard to call it an act of creation by either. It cannot have two independent creators at two different points in time and space, I would say, but it can have two independent discoverers.
"Can be thought" about is extremely broad, but that's approximately how broad my ontology extends, because I hold abstract objects to exist.
From a Platonist stance, Lao Tzu, for example, is certainly real, but the label is overloaded. It refers both to a concept of a person and to a person who may not have existed who is described by that concept. The concept exists. The person may not have.
However, you are right that this forces us into cumbersome language, so it fails the utility test -- but this is not so for mathematical objects. There is no question about whether there are any actual mathematical objects in addition to the concepts, because the concepts and the mathematical objects are one and the same.
So, while I would say that Lao Tzu exists from a Platonist perspective, I would not normally adopt the Platonist perspective when discussing (potentially) fictional characters. This ties in with my view that there is no right or wrong answer on whether abstract objects exist. It depends only on which perspective you choose to adopt.
Hi,
ReplyDelete"Perhaps we don't need mathematical entities as a way to explain the usefulness of mathematical tools. I didn't say we did. I'm just noting some similarities between some things which exist (including usefulness as tools) since you want the concept of existence to be unified. But we can drop this point because you're right that we don't directly use mathematical objects to do anything."
I think that Carnap covered this - you don't need to worry about consistency as long as you define your convention at the outset.
Say mathematical objects exist if it is useful for what you are doing, but in the cases where it will cloud rather than clarify then drop the terminology.
(I posted a similar message earlier but I am not sure if it got lost - so there might be some duplication).
Forgot the link.. http://www.ditext.com/carnap/carnap.html
DeleteI'm sure I've never had a particularly original thought (original thoughts are increasingly rare after thousands of years of great philosophy), so it's not surprising to me that Carnap has expressed similar ideas.
DeleteHowever, where I think I differ from Carnap is that he thinks you can adopt this convention without committing to a Platonist ontology. On the other hand, I think that's all a Platonist ontology is. I think ontologies are nothing more than conventions for what to consider as existing and what to count as unreal. I don't think the concept of existence is a clear, objective thing that has one preferred definition.
I would also focus less on Platonism as a linguistic convention and concentrate a little more on it as a perspective or way of thinking about things.